TRANSFORMER PARAMETERS
Transformer Model
Overview: The change of voltage in a transformer between the primary and secondary is directly related to the turns ratio of the coils. Since the transformer is not able to generate power but only convert it, the change in currents is inversely related to the change in voltage.
Basic: The basic transformer model consists of a primary winding coil of turns NP and secondary winding coil of turns NS wound on a magnetic core.
Thus the output voltage (VS) and current (IS) are related to the input supply voltage (VP) and input current (IP) by the following equations.

Realistic: In reality the coils have resistances (RP & RS) associated with the conductors used to wind them. They will also have a leakage inductance associated with the number of turns and the geometry of the coils (LP & LS). There are also inductive and resistive components (LC & RC) that electrically represent the equivalent magnetic properties of the core. These parameters result in a more complex but realistic transformer model
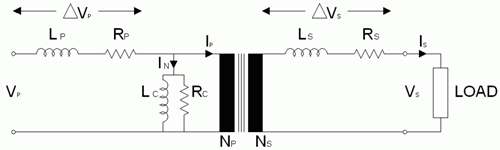
Each of these components will have an effect on the transformer performance that has to be considered in the design of the unit to ensure the final product conforms to specification.
No Load Characteristics
When there is no load connected to the transformer output, IP and IS are zero. However there is still a small current drawn by the transformer from the supply. This current is required to magnetize the core in one direction, then the opposite, as the AC supply swings through a full mains cycle. This current is known as the No Load Current “IN”
The transformer core is subject to a loss mechanism known as hysteresis loss. This is the No Load Loss or Core Loss, WCR, Although magnetic in origin can be represented electrically in the transformer model by the portion of the No Load Current that flows through the resistor RC.
Under no load conditions both IP and IS are zero thus ΔVS zero while ΔVP is only affected by IN and is consequently small. For this reason the No Load Output Voltage is given by the equation 1 from the basic transformer model
Coil Losses / Load Losses
When the transformer is on load such that the current IS flows in the load and IP in the primary circuit of the transformer. Then the coils of the transformer will dissipate power in a form associated with “Ohmic Heating”
The total coil losses will be given by
![]()
These losses apply at the room temperature. As the temperature of the transformer rises both RP and RS will increase in value.
The total transformer losses are given by the sum of the core losses and the coil losses
![]()
As transformers increase in size the current carrying area of the conductors will increase. This will result in two other coil loss mechanisms starting to appear.
Skin Depth Losses
When a current first starts to flow in a conducting wire it initially flows in the outer surface of the conductor then gradually penetrates further into the bulk of the cross-sectional area. The lowest resistance the current sees occurs once the full cross-section of the conductor is being used to carry the current.
When an alternating voltage is applied to a system a current starts to flow then stops then starts to flow again in a repetitive cycle.
If the rate at which the current starts and stops is faster than the time it takes to fully penetrate the conductor cross-section then the effective resistance of the conductor is increased and the coil losses rise
The depth to which the current can penetrate within the cycle time is known as the “Skin Depth”
Carroll & Meynell design products such that the conductor thickness remains below this critical size
Eddy Current Losses
When conductor cross-sections become large then circulating currents start to be generated within the conductor itself. These currents are usually defined as a percentage of the load current and contribute to the self heating of coils by increasing the I²R values. These currents reduce in magnitude as the temperature increases.
Eddy currents are calculated by comparing the actual measured coil losses to the theoretical I²R losses.
Harmonic Effects
Another factor that can influence the coil losses is non-linear loads which create current harmonics in the transformer windings, see K-Factor Transformers
Efficiency
The efficiency of the transformer is defined as the power it can deliver to the load as a percentage of the total power drawn from the supply i.e. the load power plus the transformer losses. The efficiency is usually expressed as a percentage
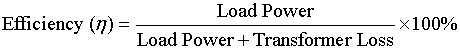
| Transformer Rating |
100VA
|
250VA
|
500VA
|
1kVA
|
10kVA
|
| Typical Efficiency |
80%
|
89%
|
92%
|
94%
|
97%
|
Regulation
As the load on a transformer is varied, the output voltage also changes. The extent of this change is known as the regulation.
As the load changes the variation in currents IP and IS cause changes in the voltage drop across the coil impedances ΔVP and ΔVS. The regulation, expressed as a percentage, is defined for the change in VS from No Load to Full Load

Carroll & Meynell design transformers compensating for the effects of regulation to give the correct output voltage at full load.
| Transformer Rating |
100VA
|
250VA
|
500VA
|
1kVA
|
10kVA
|
| Typical Regulation |
12%
|
9%
|
5%
|
4%
|
1%
|
Impedance
The resistances and inductances of the transformer windings make up the impedance.
If we apply a direct short circuit to the output of the transformer then IS will attempt to increase to an infinite value. In reality ISwill be limited by the impedances of the coils, RP, RS, LP and LS.
The impedance of the transformer is measured by placing a direct short circuit on the output of the transformer then gradually increasing an input test voltage from 0Volts to a value VI whereby the short circuit current flowing in the transformer coils equals nominal rated load currents of the transformer. This voltage VI is referred to as the impedance voltage and is expressed as a percentage of the nominal rated input voltage
![]()
The short circuit fault current can then be calculated as

| Transformer Rating |
100VA
|
250VA
|
500VA
|
1kVA
|
10kVA
|
| Typical Impedance |
10%
|
8%
|
5%
|
4%
|
1.5-2%
|
| Typical Fault Current |
10x
|
12x
|
20x
|
25x
|
50-65x
|
